Critical Magnetic Field

The superconducting state cannot exist in the presence of a magnetic field greater than a critical value, even at absolute zero. This critical magnetic field is strongly correlated with the critical temperature for the superconductor, which is in turn correlated with the bandgap. Type II superconductors show two critical magnetic field values, one at the onset of a mixed superconducting and normal state and one where superconductivity ceases.

The dependence of the critical magnetic field upon temperature is of the approximate form

Bc(T) = Bc(0)(1 - (T/Tc)2)

which shows that a magnetic field works against superconductivity.

Why does a magnetic field work against superconductivity?
Illustrate mixed state Examples

 concepts

  Go Back





Critical magnetic fields for superconductors

Type I Type II Discussion
Critical fields on periodic table
 concepts

  Go Back





Phase Diagram Examples

The Type II superconductors have much higher critical magnetic fields than Type I, but for most of that field range they are mixtures of normal and superconducting.

Illustrate mixed state
concepts

  Go Back





Vortex State for Superconductors

Type II superconductors usually exist in a vortex state with normal cores surrounded by superconducting regions. This allows magnetic field penetration. As their critical temperatures are approached, the normal cores are more closely packed and eventually overlap as the superconducting state is lost.

Further discussion of vortices

 concepts

  Go Back





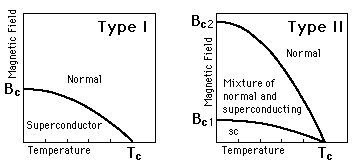
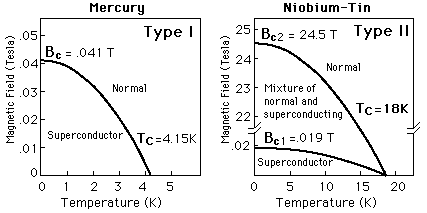
Vortex State for Superconductors

At the lower of the two critical magnetic fields in a Type II superconductor, magnetic fields begin to penetrate through cores of normal material surrounded by superconducting current vortices. As long as these vortices are stationary (pinned), the magnetic fields can penetrate while still maintaining zero electric resistivity paths through the material. A size of about 300 nm is typical for the normal cores. While the Meissner effect is modified to allow magnetic fields through the normal cores, magnetic fields are still excluded from the superconducting regions.
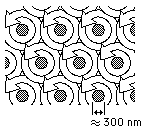As the temperature or the external magnetic field is increased, the normal regions are packed closer together. The vortices feel a force when current flows, and if they move, the superconducting state is lost. Microscopic defects can act to pin the vortices and maintain the superconducting state to a higher temperature. So the microscopic structure and fabrication techniques influence their properties greatly.

Magnetic fields do penetrate the Type-II superconductors through the normal cores in a mixed-state Meissner effect. The vortices can actually be imaged by magneto-optical techniques. The magnetic field associated with the vortices can rotate the plane of polarization of incoming linearly polarized light by the Faraday effect. With a ferrite type detector and crossed polarizers, the vortices are seen as bright spots and can be observed in real time.



 concepts

  Go Back